Links table
Abstract and 1 introduction
1.1 Esprit and expand the central limit error
1.2 Contribution
1.3 Related work
1.4 Technical Overview and 1.5 Organizations
2 Evidence of measuring the central limit error
3 Evidence of the exhausting of the optimal error
4 second -class Eigenvertor theory
5 Strong EignVECTOR
5.1 Building “Good” p
5.2 Taylor expanded regarding the terms of error
5.3 Cancel the error in Taylor’s expansion
5.4 evidence of theory 5.1
Introductory
B Vandermonde Matrice
A deficient proof of Article 2
D proofs postponed for Article 4
The postponed proofs of Article 5
F lown Lond for spectral appreciation
Reference
2 Evidence of measuring the central limit error
The main proof road map is the same as the previous work [LLF20] With small adjustments. All technical evidence and accounts are postponed to append
This result is similar to the current results such as [Moi15, Lem. 2.7] and [LLF20, Lems. 2, 5, & 6] Our proof (in the C.2 supplement) is based on similar ideas. The main components in proving this result are the borders of the moitra on the individual values of the Vandermonde matrix (appendix B.1), the standard results in the theory of archeological disorder (appendix A.5), and the LEMMA comparison between Vandermonde and eignbases (Lemma 1.7)
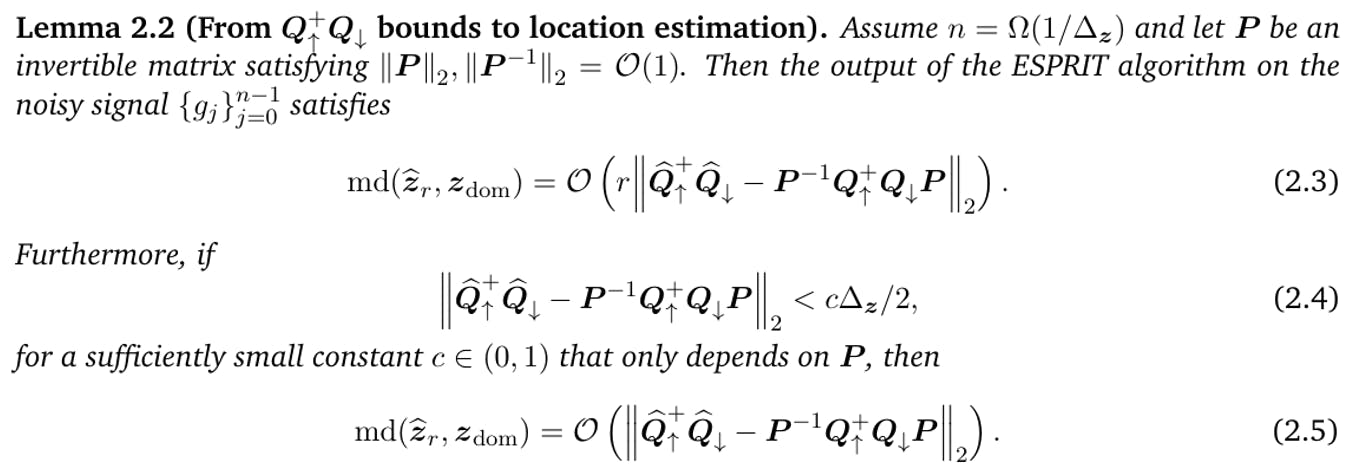
To convert the tied equation. (2.2) To ESPRITIDERIAL Infinitive Information, we use the following result:
This result is slightly improved from LEMMA 2 in [LLF20] It is proven by combining the theory of Bauer -Rike (Theorem A.11) and the appendix of the broadcaster to disturb the self -value (Lemma A.13). The evidence is postponed on the appendix C.3.
Authors:
(1) Chayan Ding, Department of Mathematics, University of California, Berkeley;
(2) Ethan n. Brace, Department of Computing and Sports Science, California Institute of Technology, Pasadina, California, USA;
(3) Lynn, Department of Mathematics, University of California, Berkeley, Department of Applied Mathematics and Accounts Research, Lawrence Berkeley National Institute, and the Institute of Challenge for quantitative account, University of California, Berkeley;
(4) Ruwaiza Chang, Simons Institute for Computing Theory.
