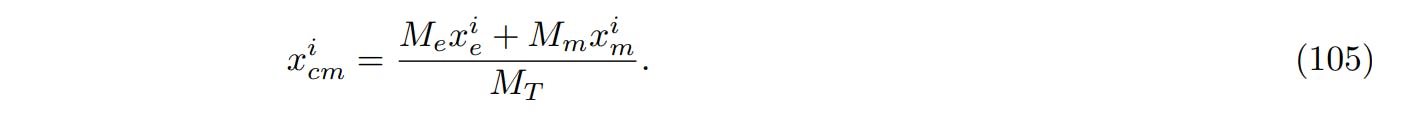Movement equations in the Earth system

Links table

Abstract and 1. Introduction

2. The watch is in orbit

   2.1 Time Format

   2.2 The domestic frame for the moon

3. Differences of the average clock between the earth and the moon

4. Watch at Lagance points on Earth

   4.1 The watch in Lagrang Point L1

   4.2. The watch is in Lagrang Point L2

   4.3. The watch is in Lagrange Point L4 or L5

5. Conclusions

Approach 1: Fermi coordination with the original in the center of the moon

Approach 2: Building the center of the bloc frame freely

Things 3: Earth and moon movement equations

Approach 4: Comparing the results in rotating and non -periodic coordinates systems

Thanks, appreciation and references

Things 3: Earth movement equations and Mo

This partial derivative should be evaluated in the center of the Earth, which would present a uniqueness. However, the body cannot cause its mass center to accelerate, so the term that involves the capabilities of the Earth should be “must be” or ignored. Then it becomes an equation of the movement of the earth

An argument similar to the moon equation gives

The center of the Earth’s system mass should be in

Take the linear plans corresponding to the above equations of the movement given

Thus checking that the center of the Earth’s system mass is not accelerated in this coordinate system.

Let the head from the center of the earth to the center of the moon indicate it D. Then he took the difference between the two equals above the movement

Where the distance between the Earth and the moon is given by EQ. (19). then

The equivalent land system satisfies the Kepler equation in the Earth’s Earth’s Earth Earth plane:

In short, we built a reference frame with a fall of the fall maritime with the original in the middle of the land and moon, and we showed that the Earth and the moon revolve around the center of the mutual mass in the orbit of Keplerian. Parties are not natural coordinates Fermi, meaning that the second type Christopheel symbols are not zero in the origin of coordinates when they are calculated in these coordinates. This is because the geodesy, which does not explain the original, is strong on a test particle originally because of the Earth and the moon only because of the sun.